Math problems about our Solar System
Mercury
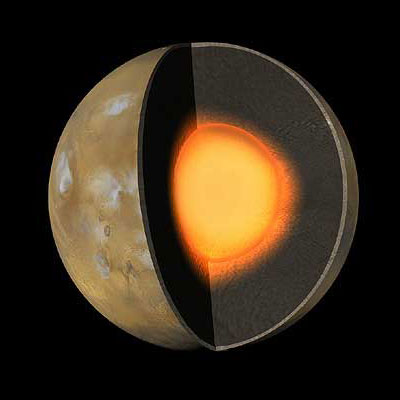
Problem 474: MESSENGER Explores the Interior of Mercury Students work with a simple spherical core and shell model to determine the interior structure of mercury and the size of its dense iron core. [Grade: 9-12 | Topics: working with volumes of speheres; mass = density x volume] [Click here]
Problem 473: MESSENGER Explores the Mass of Mercury Students use the orbit of NASA's MESSENGER spacecraft to determine the mass of Mercury. [Grade: 9-12 | Topics: working with equations with integer powers and solving for specified values; scientific notation] [Click here]
Problem 415: Mercury and the Moon - Similar but different Students explore the mass and volume of mercury compared to the moon by using the formula for a sphere and scale changes. [Grade: 8-10 | Topics: scale; volume of a sphere; mass = density x volume] [Click here]
Problem 121: Ice on Mercury? Since the 1990's, radio astronomers have mapped Mercury. An outstanding curiosity is that in the polar regions, some craters appear to have 'anomalous reflectivity' in the shadowed areas of these craters. One interpretation is that this is caused by sub-surface ice. The MESSENGER spacecraft hopes to explore this issue in the next few years. In this activity, students will measure the surface areas of these potential ice deposits an calculate the volume of water that they imply. [Grade: 8-10 | Topics:Area of a circle; volume, density, unit conversion] [Click here]
Problem 105: The Transit of Mercury As seen from Earth, the planet Mercury occasionally passes across the face of the sun; an event that astronomers call a transit. From images taken by the Hinode satellite, students will create a model of the solar disk to the same scale as the image, and calculate the distance to the sun. [Grade: 9-11 | Topics:image scales; angular measure; degrees, minutes and seconds] [Click here]
Venus
Mars
Problem 634: History of Winter - What is a Snowballs Chance on Mars? Students explore the phase diagrams for water and carbon dioxide and discover whether astronauts would be able to create snowballs on mars made from carbon dioxide ice. [Grade: 9-12 | Topics: Graph analysis] [Click here]
Problem 633: History of Winter - Exploring Temperature and States of Matter Students learn how to read a simple phase diagram and how states of matter are related to temperature and pressure. [Grade: 9-12 | Topics: Rates of Change; Unit conversions; decimal math ] [Click here]
Problem 632: History of Winter - Exploring Energy and Temperature Students learn about the relationship between temperature and the kinetic energy of particles. [Grade: 9-12 | Topics: Evaluating equations] [Click here]
Problem 615: Radiation Levels on the Surface of Mars Students explore radiation dosages on mars and in interplanetary space [Grade: 6-8 | Topics: unit conversions; graph analysis; rates ] [Click here]
Problem 570: Curiosity Heads for Mt Sharp Tabular data is used to estimate how long it will take the Curiosity rover to reach the base of Mt Sharp using data from its previous-week travels. [Grade: 3-5 | Topics: averaging numbers in a table; time = distance/speed] [Click here]
Problem 536:Exploring a Possible InSight Landing Area on Mars Students work with latitude and longitude and scaled images of mars to locate the InSight proposed landing area, and describe the terrain of the landing area. [Grade: 6-8 | Topics: degree measure; latitude and longitude; working with scaled images; metric measure] [Click here]
Problem 535:Comparing the InSight Landing Area to a City Block! Students use scaled images of a proposed InSight landing area and a scaled image of an urban neighborhood on Earth to compare the sizes of familiar things with the unfamiliar martian landscape. [Grade: 6-8 | Topics: scale; proportion; metric measurement] [Click here]
Problem 534:Exploring Marsquake Energy with the Moment Magnitude Scale Students are introduced to the Moment Magnitude marsquake scale which gives a logarithmic index for marsquakes of differing energies. They calculate two examples of marsquakes and meteor impacts and compare their Moment Magnitude. [Grade: 8-10 | Topics: logarithms; scientific notation; algebra ] [Click here]
Problem 533:Exploring Logarithms and the Richter Magnitude Scale Students work with a logarithmic scale to estimate how much ground movement occurs for earthquakes of different strengths. [Grade: 8-10 | Topics: logarithms; base-ten exponents] [Click here]
Problem 532:The Distance to the Martian Horizon Students devive a basic equation for the distance to the horizon on a spherical body using the Pythagorean Theorem and a bit of algebra. The estimate the number of cell towers needed to cover Mars. [Grade: 8-10 | Topics: Pythagorean Theorem, Algebra; scientific notation; areas of spheres and circles ] [Click here]
Problem 531:Exploring the Interior of Mars with Spheres and Shells Students use the volume properties of spheres and shells along with the relationship mass=densityxvolume to create a model of the interior of mars. [Grade: 8-10 | Topics: formula for volume of spheres and spherical shells; mass=densityxvolume; scientific notation ] [Click here]
Problem 530:Exploring the Mass of Mars Students calculate the mass of mars by using satellite data and Keplers Third Law. [Grade: 8-10 | Topics: Algebra; scientific notation ] [Click here]
Problem 529:Exploring Impacts and Quakes on Mars Students work with logarithmic scales to explore the relationship between the energy of an marsquake and its logarithmic index, which is similar to the Richter Scale used for earthquakes. [Grade: 8-10 | Topics: Logarithmic scales; scientific notation ] [Click here]
Problem 528:Comparing the Heat Output of Mars and Earth Students learn about the heat flow formula and use it to explore the properties of Earth and Mars in terms of their crust composition. [Grade: 8-10 | Topics: Algebra; temperature gradients] [Click here]
Problem 527:Exploring Heat Flow and Insulation Students explore how insulation works to reduce heat flow. They convert a verbal description of a formula expressed in proportions, and use it to calculate why aluminum pots heat faster than steel pots, and how we can determine the properties of martian soil from heat flow and temperature changes. [Grade: 8-10 | Topics: algebra; rates of change ] [Click here]
Problem 526:Exploring Temperature Change in Earth's Outer Crust Students explore the rate of temperature change in the crust of Earth and Mars and learn about units expressed as degrees C/km. They calculate how hot the ground will be at various depths, and how gold miners must deal with extreme heat. [Grade: 6-8 | Topics: fahrenheit and celsius degrees; rates of change] [Click here]
Problem 525:Exploring the InSight Lander Telemetry Data Flow Students explore how long it takes to transmit digital data using examples from downloading songs from their computer to their ipod. [Grade: 6-8 | Topics: working with kilo, mega and rates of data transfer in bytes/sec. ] [Click here]
Problem 524:Seeing the Martian Surface with IDC Students learn about the IDC camera and calculate resolution and how many images are needed to map the InSight landing area. [Grade: 6-8 | Topics: ANgular measurfe, degrees and seconds; image scal; tiling an area with overlap. ] [Click here]
Problem 523:Telling Time on Mars - Earth Days and Mars Sols Students work with two clocks on Earth and Mars and learn about earth and mars time given that a day on Mars is 40 minutes longer than an Earth day. [Grade: 6-8 | Topics: time calculations, hours, minutes, seconds; length of day ] [Click here]
Problem 522:Radio Communications with Earth � The Earth-Sun Angle The earth-sun angle is given in tabular form in degrees. Students graph the data and find the dates when transmissions to Earth cannot occur. [Grade: 8-10 | Topics: Interpreting tabular data; rates and slopes ] [Click here]
Problem 521:Estimating the Mass of a Martian Dust Devil! Students estimate the mass of a martian dust devil using the approximation that it is a cylinder with a fixed density of dust. [Grade: 8-10 | Topics: Volume of a cylinder; mass = density x volume ] [Click here]
Problem 520:The Work Area In Front of the Lander Students estimate the area in front of the InSight lander where experiments will be conducted and instruments moved with a single robotic arm. [Grade: 6-8 | Topics: Area of a circle segment; Area common to two intersecting circles] [Click here]
Problem 519:Scheduling Events in Time for Launch Students learn about scheduling many events along a timeline (breakfast, packing, driving, etc ) by planning a family trip where the family members have to arrive at the airport for a flight that leaves at a specific date and time. [Grade: 5-7 | Topics: working with time units; creating a timeline] [Click here]
Problem 518:The InSight Seismographic Station Solar Power System Students explore the properties of decagons to determine the area of the solar panels used on the InSight lander. [Grade: 7-9 | Topics: area of regular polygons; estimating areas of non-square shapes] [Click here]
Problem 508: The InSight Seismographic Station - Wave arrival times Students work with the circumference of Mars and the speed of shock waves in the martian crust to estimate the arrival times of the waves at the InSight Lander. [Grade: 6-8 | Topics: speed=distance/time; Time calculations; circumference of a circle] [Click here]
Problem 500: Curiosity Uses X-Ray DIffraction to Identify Minerals on Mars Students learn about diffraction geometry and then estimate the distance between crystal planes in a mars rock sample. [Grade: 10-12 | Topics: geometry; trigonometry] [Click here]
Problem 491: The Curiosity Rover on the Move. Students plot the position of the Curiosity Rover on a cartesian grid covering the satellite image of the landing area. They use the 2-point distance formula to determine how far the rover traveled between stops, and determine it speed. [Grade: 6-8 | Topics: Cartseian graphs; ordered pairs and coordinates; distance = speed x time; metric measure ] [Click here]
Problem 485: Curiosity Discovers Ancient Mars River Students estimate the speed of an ancient mars river using images from the Curiosity rover. [Grade: 9-12 | Topics: Algebra; trigonometry; evaluating functions ] [Click here]
Problem 479: Exploring Gale Crater with the Curiosity Rover Students explore the Gale Crater landing area and calculate rover distances to various way stations to determine the round trip distance and travel time. [Grade: 9-12 | Topics: Pythagorean Distance Formula; Coordinate geometry ] [Click here]
Problem 457: The Interplanetary Voyage of MSL Students use the properties of ellipses to determine the formula for the Hohmann Transfer Orbit taking the Mars Science Laboratory to Mars in 2012 [Grade: 10-11 | Topics: time=distance/speed; scale models; metric math; properties of ellipses] [Click here]
Problem 456: The Launch of the Mars Science Laboratory (MSL) in 2011 Students use a sequence of launch images to determine the Atlas V's launch speed and acceleration. By determining the scale of each image, they estimate average speeds during the first 4 seconds after lift-off. [Grade: 8-10 | Topics: time=distance/speed; scale models; metric math] [Click here]
Problem 393: Taking a stroll around a martian crater! Students use a recent photograph of a crater on Mars to estimate its circumference and the time it will take NASAs Opportunity Rover to travel once around its edge. [Grade: 6-8 | Topics: scale model; distance = speedxtime; metric measure] [Click here]
Problem 237: The Martian Dust Devils Students determine the speed and acceleration of a martian dust devil from time laps images and information about the scale of the image. [Grade: 6-8 | Topics: scales; Determining speed from sequential images; V = D/T [Click here]
Problem 139: How Big Is It? - Mars Students use an image of a crater wall on mars to investigate ancient water gullies discovered in 2008 by the Mars Orbiter. [Grade: 4 - 7 | Topics:image scales; metric measurement; division and multiplication; decimals] [Click here]
Problem 133: How Big is It? - The Mars Rover. Students work with an image taken by the Mars Orbiter satellite of the Spirit landing site. They determine the image scale, and calculate the sizes of various surface features from the image. [Grade: 4 - 7 | Topics:image scaling; multiply, divide, work with millimeter ruler] [Click here]
Problem 126 : How Big is It? - A Martian Avalanche! Students work with a Mars reconnissance Orbiter image to determine image scale, and search for the smallest things seen in a photograph.This avalanche was caught as it occurred on February 19, 2008! [Grade: 4 - 7 | Topics:image scaling; multiply, divide, work with millimeter ruler] [Click here]
Problem 74: A Hot Time on Mars - The NASA Mars Radiation Environment (MARIE) experiment has created a map of the surface of mars, and measured the ground-level radiation background that astronauts would be exposed to. This math problem lets students examine the total radiation dosage that these explorers would receive on a series of 1000 km journeys across the martian surface. The students will compare this dosage to typical background conditions on earth and in the International Space Station to get a sense of perspective [Grade level: 6-8 | Topics: decimals, unit conversion, graphing and analysis ] [Click here]
Problem 70: Calculating Total Radiation Dosages at Mars - This problem uses data from the Mars Radiation Environment Experiment (MARIE) which is orbiting Mars, and measures the daily radiation dosage that an astronaut would experience in orbit around Mars. Students will use actual plotted data to calculate the total dosage by adding up the areas under the data curve. This requires knowledge of the area of a rectangle, and an appreciation of the fact that the product of a rate (rems per day) times the time duration (days) gives a total dose (Rems), much like the product of speed times time gives distance. Both represent the areas under their appropriate curves. Students will calculate the dosages for cosmic radiation and solar proton flares, and decide which component produces the most severe radiation problem. [Grade level: 6-8 | Topics: decimals, area of rectangle, graph analysis] [Click here]
Jupiter
Problem 568: Ios Volcanoes and Resurfacing Students examine how volcanic activity on Jupiters satellite Io can lead to fresurfacing the entire moon in less than a million years covering all new craters. [Grade: 6-8 | Topics: Surface aea of a sphere; rates; scientific notation] [Click here]
Problem 472: Investigating Juno's Elliptical Transfer Orbit Students use the Standard Formula for an ellipse to study the elliptical orbit of the Juno spacecraft, and relate specific properties of the ellipse to features of the spacecrafts trajectory such as aphelion, perihelion, and ellipticity. [Grade: 9-12 | Topics: formula for an ellipse; semi-major and minor axis] [Click here]
Problem 471: Investigating the Launch of the Juno Spacecraft Students use a series of images from a launch video to determine the scale of each image and determine the speed of the rocket as it leaves the gantry. [Grade: 6-8 | Topics: scale models; speed = distance/times] [Click here]
Problem 470: The Launch of the Juno Spacecraft - Ascent to orbit Students use tabulated altitude and range data following the launch of the Juno mission, to determine the speed of the rocket as it travels from the ground to earth orbit. [Grade: 6-8 | Topics: scale models; speed = distance/time] [Click here]
Problem 469: Solar Energy and the Distance of Juno from the Sun Students use the formula for an ellipse, along with the inverse-square law to create a mathematical model that predicts the declining solar power produced by Junos solar panels as the spacecraft travels from Earth to Jupiter. [Grade: 9-12 | Topics: algebra; trigonometry; distance formula] [Click here]
Problem 135 How Big is It? - Io and Jupiter. Students work with an image taken by the Cassini spacecraft of Jupiter and its satellite Io. They determine the image scale, and calculate the sizes of various features in the image. [Grade: 4 - 7 | Topics:image scaling; multiply, divide, work with millimeter ruler] [Click here] [Click here]
Saturn
Problem 572: How Saturns Moon Mimas Created the Cassini Division Students calculate the acceleration of gravity in Cassinis Division and estimate the number of years to eject these particles. [Grade: 9-12 | Topics: scientific notation; evaluating a formula for gravity; unit conversions] [Click here]
Problem 569: Orbit Speeds and Times for Saturns Rings Students learn about the orbit speeds of ring particles and how orbit periods in the Cassini Division relate to the orbit of the moon Mimas. [Grade: 6-8 | Topics: square root formulae; circumference of circle; speed = distance/time ] [Click here]
Problem 550:Comparing the Rings of the Outer Planets Students compare the dimensions of the rings of Jupiter, Saturn, Uranus and Neptune to the radius of each planet, and the location of the break up Tidal Limit to test an idea of how the rings may have formed. [Grade: 6-8 | Topics: scale model; proportions; number line ] [Click here]
Problem 549:Saturns Rings- Shadows from Moons and Ringlets Students use an image of a ring of Saturn to investigate its thickness using shadows cast by ringlet material kicked up by a passing moon. [Grade: 6-8| Topics: scales; proportions; triangle geometry; angle measurement] [Click here]
Problem 548:Saturns Rings - A close-up study Students use a Cassini image of Saturns rings to calculate the sizes of the smallest rings, and how their thicknesses change with distance from Saturn. [Grade: 3-5 | Topics: measurement; scales; proportions; metric measure; bar graphs] [Click here]
Problem 547:The Rings of Saturn Students explore the volume and mass of the rings of Saturn to estimate the number of ring particles and their separations, and the radius of the equivalent spherical body. [Grade: 9-12 | Topics: volume of a ring and a sphere; scientific notation] [Click here]
Problem 461: Cassini Delivers Holiday Treats from Saturn Students explore proportions and angular size using images of Saturn's moons Titan and Dione [Grade: 7-9 | Topics: scale models; proportions] [Click here]
Problem 335: Methane Lakes on Titan Students use a recent Cassini radar image of the surface of Titan to estimate how much methane is present in the lakes that fill the image, and compare the volume to that of the fresh water lake, Lake Tahoe. [Grade: 6-8 | Topics: estimating irregular areas; calculating volume from area x height; scaled images ] [Click here]
Problem 315: The Mysterious Hexagon on Saturn A curious hexagon formed by the Saturn polar jet stream, and photographed by the Cassini spacecraft, is used to determine wind speed and acceleration. [Grade: 6-9 | Topics: Measuring; Metric Units; speed=distance/time] [Click here]
Problem 272: Spitzer Telescope Discovers New Ring of Saturn! Students calculate the volume of the ring and compare it to the volume of Earth to check a news release figure that claims 1 billion Earths could fit inside the new ring. [Grade: 8-9 | Topics: Geometry, Algebra, volumn, scientific notation] [Click here]
Problem 205: The Io Plasma Torus- Students approximate the Io radiation belts as a cylinder to determine its volume ,and the mass of the particles within it. [Grade: 9-12| Topics: Algebra I - volume of cylinders; calculus - Integrals of volumes.] [Click here]
Problem 174: A Matter of Timing - Students study satellites of Saturn to work out graphically how often they will line up. [Grade: 3-6| Topics: scale model; time calculation; fractions; working with rulers and compasses] [Click here]
Problem 154: Pan's Highway and Saturn's Rings - Students use an image from the Cassini spacecraft to determine how large the satellite Pan is, and the scale of Saturn's rings using a millimeter ruler. [Grade: 4-6 | Topics:Finding the scale of an image; measurement; unit conversion] [Click here]
Uranus and Neptune
Dwarf Planets
Exploring the Dwarf Planets Scientists can learn a lot about the inside of a dwarf planet by making very precise measurements of its diameter and mass. From these measurements, average densities (mass divided by volume) can be figured out. The density of an object gives us a clue as to whether it is mostly rocky or mostly icy. [Grade: 6-8 | Topics: density; mass] [Click here]
Exploring the Dwarf Planet Ceres Counting and measuring craters on Ceres can provide insights into the cratering process that created its surface. It appears to have fewer large craters than scientists had expected to see, a possible indication that most of the material that came together to form Ceres was smaller asteroids. [Grade: 6-8 | Topics: scale; proportion] [Click here]
Triton: The Twin of Pluto? Triton is only slightly larger than Pluto. Both worlds have similar surface materials, such as nitrogen, methane and carbon monoxide. Their diameters, masses and densities are amazingly similar. [Grade: 6-8 | Topics: volume=area x height; rates;volume of a sphere] [Click here]
The Amazing Journey to Pluto! Deep space missions can take up to 10 years from development to launch. For New Horizons, it took close to 20 years from the time that scientists conceived of the mission to the time it reached its destination! [Grade: 6-8 | Topics: speed; time; unit conversion] [Click here]
Exploring Interplanetary Communication On July 14, 2015, NASA’s New Horizons spacecraft reaches dwarf planet Pluto and begins sending data back to Earth. At that time, the distance from Earth to Pluto is 4.8 billion kilometers. At the speed of light, one-way radio signal travel time is 16,000 seconds or 4 hours and 27 minutes. [Grade: 6-8 | Topics: distance; speed; time] [Click here]
Problem 605: The Solar System Beyond the Orbit of Neptune Students compute the volume and density of the Kuiper Belt located beyond the orbit of Neptune, and estimate how far apart the objects are located compared to the earth-sun distance. [Grade: 9-12 | Topics: volume of a disk; density = number/volume ] [Click here]
Problem 60 When is a planet not a planet? - In 2003, Dr. Michael Brown and his colleagues at CalTech discovered an object nearly 30% larger than Pluto, which is designated as 2003UB313. It is also known unofficially as Xenia, after the famous Tv Warrior Princess! Is 2003UB313 really a planet? In this activity, students will examine this topic by surveying various internet resources that attempt to define the astronomical term 'planet'. How do astronomers actually assign names to astronomical objects? Does 2003UB313 deserve to be called a planet, or should it be classified as something else? What would the new classification mean for asteroids such as Ceres, or objects such as Sedna, Quaoar and Varuna? [Grade level: 6-8 | Topics: Non-mathematical essay; reading to be informed] [Click here]
General Solar System Problems
Problem 555:Exploring Your Weight Across the Solar System Students estimate their weight on different planets, moons and asteroids. [Grade: 6-8 | Topics: proportions] [Click here]
Problem 554:Exploring Volcanoes and Geysers Across the Solar System Students determine the ejection speed and heights of gasses vented by geysers and volcanoes. [Grade: 6-8 | Topics: solving square root equations; ] [Click here]
Problem 546: The Relative Sizes of Planets and other Objects Students use proportional information to determine the relative scales of planets and large moons across the solar system. [Grade: 3-5 | Topics:scale; proportion] [Click here]
Problem 545:Measuring Atmospheric Trace Gases Using Parts Per Million Students convert from percentage units to parts per million and compare trace gases in the atmospheres of various planets. [Grade: 6-8 | Topics: percentages; unit conversions ] [Click here]
Problem 544:The Composition of Planetary Atmospheres Students study the composition of planetary atmospheres and compare the amounts of certain compounds in them [Grade: 6-8 | Topics: Pie graphs; percentages; scientific notation] [Click here]
Problem 543:Timeline for Planet Formation Students calculate time intervals in millions and billions of years from a timeline of events [Grade: 3-5 | Topics: time calculations; integers] [Click here]
Problem 542:The Late Heavy Bombardment Era Students estimate the average arrival time of large asteroids that impacted the moon. They work with the formula for the volume of a sphere to estimate how much additional mass was added to the moon and Earth durung this era. [Grade: 6-8 | Topics: volume of spheres; proportions] [Click here]
Problem 541:How to Build a Planet Students study planet growth by using a clay model of planetessimals combining to form a planet by investigating volume addition with spheres. [Grade: 3-5 | Topics: graphing; counting] [Click here]
Problem 540:Travel Times by Spacecraft Around the Solar System Students explore how long it takes our fastest rockets to reach each of the planets. [Grade: 6-8 | Topics: time=distance/speed; metric conversion] [Click here]
Problem 539:Visiting the Planets at the Speed of Light Students learn about the light travel times to the 8 planets by converting the distances in Astronomical Units to travel times at the speed of light. [Grade: 6-8 | Topics: Proportions; unit conversions; time = distance/speed; metric units] [Click here]
Problem 538:How Big is Our Solar System? Students work with proportions to convert solar system distances into Astronomical Units for the 8 planets. [Grade:6-8 | Topics: Proportions; unit conversions] [Click here]
Problem 496: How to Grow a Planet or a Rain Drop Students use calculus to slove for the growth in mass of a body, and solve the equation for the case of a raindrop and a planet like Earth. [Grade: 12 | Topics: Solving a simple differential equation.] [Click here]
Problem 464: Big Moons and Small Planets Students work with a scaled drawing of 26 large moons in the solar system, and together with an exercise in using simple fractions, explore the relative sizes of the moons compared to Earth. [Grade: 3-5 | Topics: scale models; proportions; fractions] [Click here]
Problem 305: From Asteroids to Planetoids Students explore how long it takes to form a small planet from a collection of asteroids in a planet-forming disk of matter orbiting a star. [Grade: 11-12 | Topics: Integral calculus] [Click here]
Problem 304: From Dust Balls to Asteroids Students calculate how long it takes to form an asteroid-sized body using a simple differential equation. [Grade: 11-12 | Topics: Integral Calculus] [Click here]
Problem 303: From Dust Grains to Dust Balls Students create a model of how dust grains grow to centimeter-sized dust balls as part of forming a planet. [Grade: 11-12 | Topics: Integral Calculus] [Click here]
Problem 302: How to Build a Planet from the Inside Out Students model a planet using a spherical core and shell with different densities. The goal is to create a planet of the right size, and with the correct mass using common planet building materials. [Grade: 9-11 | Topics: Geometry; volume; scientific notation; mass=density x volume] [Click here]
Problem 301: Planetary Alignments Students combine a geometric model with number series to calculate when planets will 'line up' in a simple solar system. [Grade: 4-8 | Topics: Number series; geometry; Least Common Multiple] [Click here]
Problem 299: Changing Perspectives on the Sun's Diameter Students compare two images of the sun taken by the SHOH satellite to measure the apparent diameter change from different earth obit locations in the winter and summer. [Grade: 6-8 | Topics: measurement; parallax; metric units; percentage change] [Click here]
Problem 296: Getting an Angle on the Sun and Moon Students explore angular size and scale by comparing two images of the sun and moon which have identical angular size, but vastly different scales. [Grade: 8-10 | Topics: Geometry; angle measure; scale; proportion] [Click here]
Problem 292: How Hot is That Planet? Students use a simple function to estimate the temperature of an exoplanet called CoRot-7b. [Grade: 8-10 | Topics: Algebra II; Evaluating Power functions] [Click here]
Problem 278: Spitzer Studies the Distant Planet Osiris Students learn about the density of the planet HD209458b, also called Osiris, and compare it to that of Jupiter. [Grade: 8-10 | Topics: Spherical volumes; density; Scientific Notation;] [Click here]
Problem 268: Planetary Conjunctions Students study a simple solar system with three planets and work out how often planets will 'line up'. [Grade: 3-5 | Topics: geometry, time, patterns] [Click here]
Problem 267: Identifying Materials by their Reflectivity The reflectivity of a material can be used to identify it. This is important when surveying the lunar surface for minerals, and also in creating 'green' living environments on Earth. [Grade: 6-8 | Topics: percentage, interpreting tabular data, area ] [Click here]
Problem 264: Water on Planetary Surfaces Students work with watts and Joules to study melting ice. [Grade: 8-10 | Topics: unit conversion, rates] [Click here]
Problem 263: Ice or Water? Whether a planetary surface contains ice or liquid water depends on how much heat is available. Students explore the concepts of Specific heat and Latent Heat of Fusion to better understand the and quantify the energy required for liquid water to exist under various conditions. [Grade: 8-10 | Topics: unit conversion, scientific notation] [Click here]
Problem 250: The Most Important Equation in Astronomy Students learn about how an instrument's ability to see details depends on its size and its operating wavelength - the key to designing any telescope or camera. [Grade: 8-10 | Topics: geometry, angle measure, scientific notation] [Click here]
Problem 249: Spotting an Approaching Asteriod or Comet Students work with a fundamental equation for determing the brightness of an asteroid from its size and distance from Earth. [Grade: 10-12 | Topics: Algebra 1, logarithms, area, scientific notation] [Click here]
Problem 247: Space Mobile Puzzle Students calculate the missing masses and lengths in a mobile using the basic balance equation m1 x r1 = m2 x r2 for a solar system mobile. [Grade: 8-10 | Topics: metric measur, algebra 1, geometry] [Click here]
Problem 241: Angular Size and Similar Triangles A critical concept in astronomy is angular size, measured in degrees, minutes or arc-seconds. This is a review of the basic properties of similar triangles for a fixed angle. [Grade: 8-10 | Topics: geometry, similar triangles, proportions] [Click here]
Problem 212: Finding Mass in the Cosmos- Students derive a simple formula, then use it to determine the masses of objects in the universe from the orbit periods and distances of their satellites. [Grade: 9-12| Topics: Scientific Notation; Algebra II; parametric equations] [Click here]
Problem 203: Light Travel Times- Students determine the time it takes light to reach various objects in space. [Grade: 6-8| Topics: Scientific Notation; Multiplication; time = distance/speed.] [Click here]
Problem 180: Planets, Fractions and Scales- Students work with relative planet comparisons to determine the actual sizes of the planets given the diameter of Earth. [Grade: 4-6| Topics: scale models; decimals; fractions] [Click here]
Problem 114 The Heliopause...a question of balance Students will learn about the concept of pressure equilibrium by studying a simple mathematical model for the sun's heliopause located beyond the orbit of Pluto. They will calculate the distance to the heliopause by solving for 'R' and then using an Excel spreadsheet to examine how changes in solar wind density, speed and interstellar gas density relate to the values for R. [Grade: 8-10 | Topics: Formulas with two variables; scientific notation; spreadsheet programming] [Click here]
Problem 89 Atmospheric Shielding from Radiation- III - This is Part III of a 3-part problem on atmospheric shielding. Students use exponential functions to model the density of a planetary atmosphere, then evaluate a definite integral to calculate the total radiation shielding in the zenith (straight overhead) direction for Earth and Mars. [Grade level: 11-12 | Topics: Evaluating an integral, working with exponential functions] [Click here]
Problem 88 Atmospheric Shielding from Radiation- II - This is the second of a three-part problem dealing with atmospheric shielding. Students use the formula they derived in Part I, to calculate the radiation dosage for radiation arriving from straight overhead, and from the horizon. Students also calculate the 'zenith' shielding from the surface of Mars. [Grade level: 9-11 | Topics: Algebra I; evaluating a function for specific values] [Click here]
Problem 87 Atmospheric Shielding from Radiation- I - This is the first part of a three-part problem series that has students calculate how much radiation shielding Earth's atmosphere provides. In this problem, students have to use the relevant geometry in the diagram to determine the algebraic formula for the path length through the atmosphere from a given location and altitude above Earth's surface. [Grade level: 9-11 | Topics: Algebra II, trigonometry] [Click here]
Problem 84 Beyond the Blue Horizon - How far is it to the horizon? Students use geometry, and the Pythagorean Theorem, to determine the formula for the distance to the horizon on any planet with a radius, R, from a height, h, above its surface. Additional problems added that involve calculus to determine the rate-of-change of the horizon distance as you change your height. [Grade level: 9-11 | Topics: Algebra, Pythagorean Theorem; Experts: DIfferential calculus) ] [Click here]
Problem 60 When is a planet not a planet? - In 2003, Dr. Michael Brown and his colleagues at CalTech discovered an object nearly 30% larger than Pluto, which is designated as 2003UB313. It is also known unofficially as Xenia, after the famous Tv Warrior Princess! Is 2003UB313 really a planet? In this activity, students will examine this topic by surveying various internet resources that attempt to define the astronomical term 'planet'. How do astronomers actually assign names to astronomical objects? Does 2003UB313 deserve to be called a planet, or should it be classified as something else? What would the new classification mean for asteroids such as Ceres, or objects such as Sedna, Quaoar and Varuna? [Grade level: 6-8 | Topics: Non-mathematical essay; reading to be informed] [Click here]
Problem 59 Getting A Round in the Solar System! - How big does a body have to be before it becomes round? In this activity, students examine images of asteroids and planetary moons to determine the critical size for an object to become round under the action of its own gravitational field. Thanks to many Internet image archives this activity can be expanded to include dozens of small bodies in the solar system to enlarge the research data for this problem. Only a few example images are provided, but these are enough for the student to get a rough answer! [Grade level: 6-8 | Topics: Data analysis; decimals; ratios; graphing] [Click here]
Problem 43 An Interplanetary Shock Wave On November 8, 2000 the sun released a coronal mass ejection that traveled to Earth, and its effects were detected on Jupiter and Saturn several weeks later. In this problem, students will use data from this storm to track its speed and acceleration as it traveled across the solar system. [Grade level: 6-10 | Topics: Time calculations; distance = speed x time ] [Click here]